Svenska:

Den här webbplatsen kan endast nås som arkivreferens. De officiella webbsidorna för avdelningen för partikelfysik finns på http://particlephysics.physics.lu.se

Sidan nedan slutar uppdateras fr.o.m. 2020-09-22 och uppfyller endast delvis tillgänglighetsdirektivet. Se tillgänglighetsredogörelsen

English:

The webpage you reached is made available for archiving purposes only. The official division of particle physics webpages can be found at http://particlephysics.physics.lu.se

The page below is not updated any longer since September 22, 2020 and does not conform completely to the accessibility regulation. See the accessibility statement

Total Cross section

As mentioned in section 6.4 the total cross section for $\gamma$$\gamma$ $\rightarrow$ hadrons can be extrapolated to Q² = 0 if W² > > Q_i². This is very useful when results from different experiments shall be compared. There are a number of different models to choose from, in this analysis the generalized vector meson dominance model (GVMD) was used. As seen from Fig. 8.5 this describe VSAT data well, but in the STIC Q² region it seems to be a bit low.

Figure 8.5: The Q² dependence of the total $\gamma$$\gamma$ cross section. The graph has been split in order to visualize the STIC data points.
Figure 8.6: $\sigma_{\gamma \gamma }^{}$(W²) for L3, OPAL and DELPHI VSAT experiments along with two values of the Regge parameterization.

The data points in Fig. 8.5 were obtained in the W interval from 15 to 60 GeV and clearly there is a logarithmic fall of $\sigma_{\gamma*\gamma*}^{}$(W_{$\gamma$$\gamma$}², Q₁², Q₂²). The first VSAT datapoint at a Q² of 0.09 is somewhat low, this can probably be explained by some efficiency loss in the inner edge of the detector. From the VSAT region (Q² < 1 GeV) it is possible to factorize $\sigma_{\gamma*\gamma*}^{}$ and obtain $\sigma_{\gamma \gamma }^{}$(W²) at Q² = 0. This was done for four different W intervals with equal statistics and is presented in Fig. 8.6.

Table 8.1: The total $\gamma$$\gamma$ cross section.

Q2max	$\sigma_{tot}^{\gamma\gamma}$	Q2max	$\sigma_{tot}^{\gamma\gamma}$	W$\gamma$$\gamma$	$\sigma_{tot}^{\gamma\gamma}$(Q2 = 0)
0.09	215$\pm$53	25.4	12.3$\pm$2.5	26.4	349$\pm$70
0.17	213$\pm$43	35.4	9.3$\pm$1.9	42.1	448$\pm$90
0.23	206$\pm$41	52.8	8.5$\pm$1.7	60.6	469$\pm$94
0.33	177$\pm$35	99.4	2.9$\pm$0.5	97.5	618$\pm$124

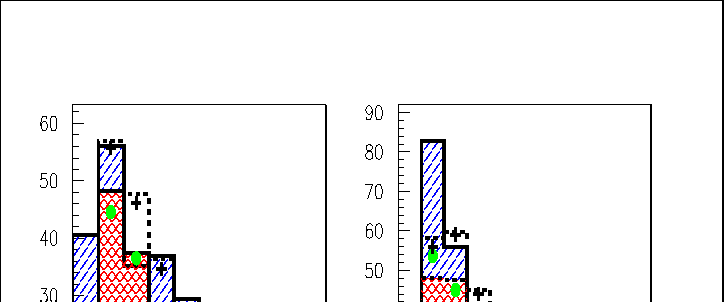
In each bin about 35 events were collected, limiting the statistical errors to about 17%. There are some systematic errors coming from the luminosity function calculation and the extrapolation of the data to Q² = 0. The uncertainty in the remaining background and some errors in Q² and W also add up to the systematical error. These are all less than 5% and are small in comparison to the statistical error. All this result in an total (systematical and statistical) error of about 20%.

The data points from OPAL [38] and L3 [39] in Fig. 8.6 were obtained by unfolding notag data with either PYTHIA or PHOJET. It is clear that VSAT data clearly favor the results obtained by PYTHIA. The two curves in Fig. 8.6 are two Regge parameterizations with different value of $\epsilon$. In the Regge theory [40] the total cross section of any hadronic process can be parameterized as:

$\sigma_{tot}^{}$ = As^$\epsilon$ + Bs^{- $\eta$}

The coefficients A and B are process and Q² dependent, whereas the values of $\epsilon$ and $\eta$ are assumed to be universal (0.093 and 0.358 respectively) [41]. If photons predominantly behave like hadrons the Regge parameterization also may be valid for the total hadronic $\gamma$$\gamma$ cross section. In Fig. 8.6 $\eta$ was fixed to 0.358 for both curves, whereas $\epsilon$ was both fitted to PYTHIA data and fixed to 0.093. In an analysis performed by the L3 collaboration a value of $\epsilon$=0.21 gives the best fit to data (for both unfolding with PHOJET and PYTHIA) and the universal value of $\epsilon$=0.093 do not describe the W dependence correctly.